Campanologomania

In an article in the Spectator in July 1711, the eponymous character Mr. Spectator—as written by Joseph Addison, one of the magazine’s founders—described his exercise routine. When in town, and therefore not able to go out riding, “I exercise myself an Hour every Morning upon a dumb Bell that is placed in a Corner of my Room, and pleases me the more because it does every thing I require of it in the most profound Silence.”^[1] We know dumbbells now as handy at-home pieces of gym equipment—free weights that have been around, in some form, at least since ancient Greek athletes used halteres to increase the length of their long jumps. But the dumbbell that Mr. Spectator refers to, and from which the heavy gym weights borrow their name, is something different. An illustration of a similar piece of equipment, published in the Gentleman’s Magazine in 1746, shows a wooden contraption in which two crossed bars with weights on the ends are mounted on an axle, around which is wound a length of rope. This mechanism would be elevated within a room, or placed in a garret, with the rope hanging down for a person standing below to pull. It mimics the apparatus used for ringing church bells, with the bell itself replaced by two weighted bars—it’s these that resemble the dumbbells of today.

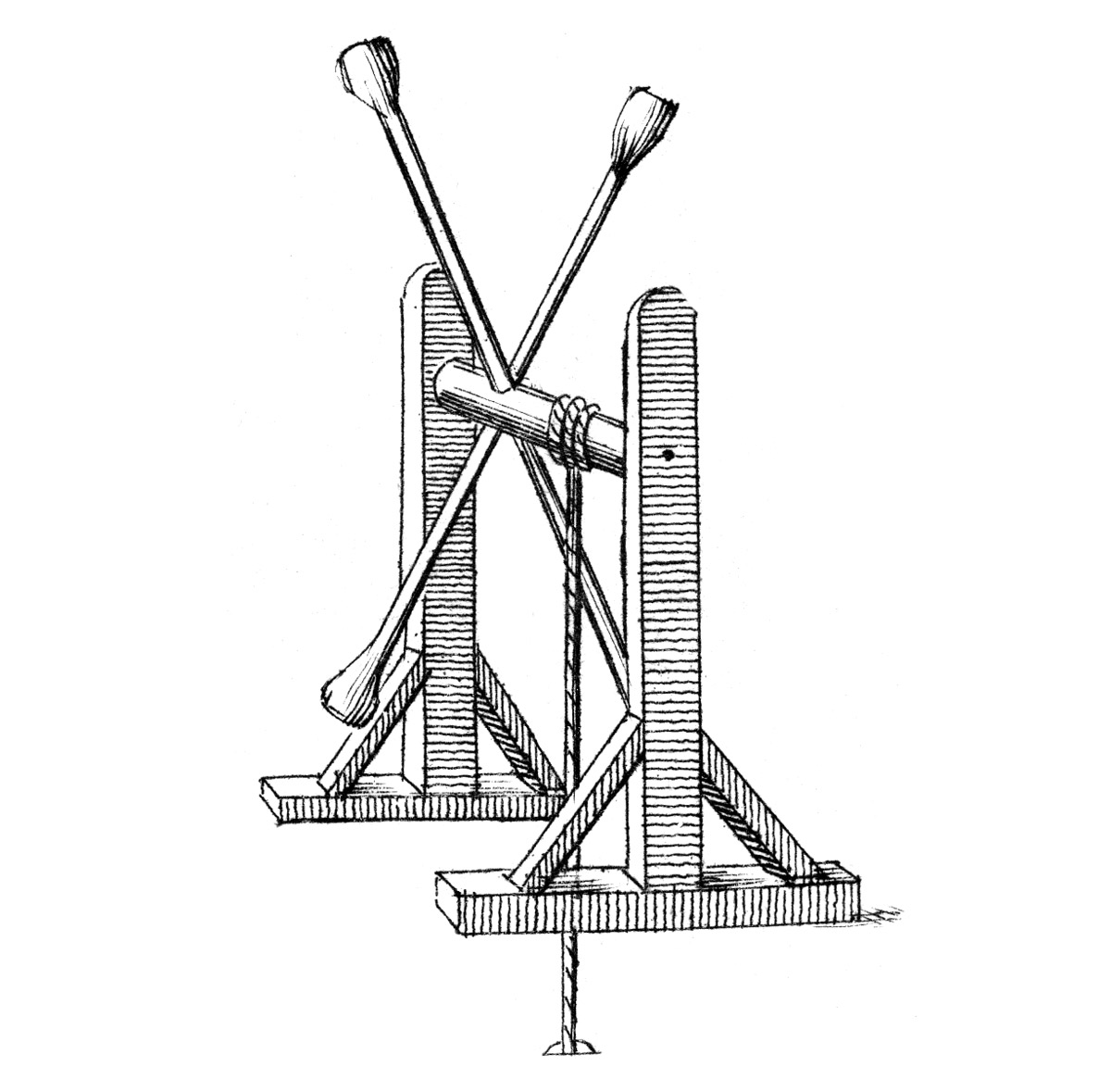
Ringing a bell, in private apartments, is here a form of quiet exercise; a church bell—a clanging, public, noisy thing—becomes, in the dumbbell, an object whose silence is its greatest asset. How did something as loud as a bell—something which is experienced so much more often, and more powerfully, by hearing than by sight—become dumb? The answer lies in the extraordinary fate of English church bells in the century before Addison was writing and, in particular, in the invention of change ringing: a technically and intellectually demanding new way of ringing bells based on the principle of constant but controlled change. Change ringing, known from the nineteenth century onward as campanology, is still practiced in England: it makes that pleasing jumble of pealing sound often used in films as an easy aural symbol of a wedding, a noise so ubiquitous that its underlying form is easily missed. In the second half of the seventeenth century, this new kind of ringing was a major craze in England, and the possibilities of its complicated system made people think about bells in a way that detached them from their older, and other, functions.

Until the middle of the sixteenth century, English church bells, like other European bells, had a variety of uses: some sacred, some secular, and many that were both. Bells called congregations to church, and told them to flee if there was a fire; they rang to signal a death in the parish, and they rang to help the passage of the souls of the dead through purgatory. Other bells, or other ways of ringing the same bells, commanded people to say a particular prayer. Bells were incredibly well-loved by their parishes and were often baptized and given godparents; their individual tones were voices that spoke to the communities over which they rang. They were among the loudest sounds in the soundscape, making up a language that its parishioners could understand.

In the Injunctions issued by the ten-year-old king Edward VI in 1547, these many and varied uses for bells were drastically reduced. Only one bell was now allowed “in convenient time to be rung or knelled before the sermon.”^[2] Bells were so useful that a single one was still to be used to call the godly to church, but in this new post-Reformation England, their other uses were no longer officially approved. The dead didn’t need help through purgatory, because it no longer existed; there was no need to command anyone to say popish prayers such as the Ave Maria by ringing the Angelus bell, because these prayers were now deemed useless. But parishioners had such affection for church bells that this particular injunction was never seriously enforced. They went to great lengths to keep their bells, sometimes by burying them until the zealous storm had passed.

What all this meant was that, despite the curtailing of their liturgical uses, at the beginning of the seventeenth century a lot of church bells remained hanging in church towers. Ringing them was an activity pursued with great enthusiasm, often by groups of boozy young men. Paul Hentzner, a German lawyer who traveled through England in the final years of the sixteenth century, wrote that the English were “vastly fond of great noises that fill the ear, such as the firing of cannon, drums, and the ringing of bells, so that it is common for a number of them, that have got a glass in their heads, to go up into some belfry, and ring the bells for hours together for the sake of exercise.”^[3] It was in these long, beer-fuelled ringing sessions that change ringing was invented, as a codification of the disorganized ringing that Hentzner describes. It seems to have started in London and southeast England in the early seventeenth century; it spread, and by the 1660s was a fashionable recreation, with societies springing up all over south, central, and eastern England to further the practice. Bells were still useful as signals, gathering and scattering the communities over which they rang; they still operated as important symbols, too. But change ringing was an innovation that overlaid, and operated in parallel with, these other signals and symbols. Whereas other Protestant countries repurposed their bells to play musical tunes, as in the carillon popular in the Netherlands, change ringing was the English answer to the question of what to do with these leftover Catholic objects.

Change ringing operates on a simple but strict system of permutations. All the bells in a church tower are rung in rounds; each ringer rings a single bell, and the bells are hung such that the ringer is able to control the sound, making the bell strike once and only once per pull. Every bell must be rung once in each round, and the order in which the bells are rung (that is, the order of the round) may never be repeated. Change-ringing notation uses numbers, with each number standing for a bell. If a ring of five bells starts ringing from highest bell to lowest, with 1 representing the highest bell and 5 the lowest, the first orderly round can be written as 12345. The following round might be 21345, then 23145, and so on; each new round is known as a change. The aim, in theory at least, is to exhaust all the possible orders in which the bells can be rung, without ever repeating a round; different ways of exhausting all the possible orders are known as “methods.” Strict rules govern which bells can swap places with which, and ringers developed a terminology—words all full of motion: bobs and dodges, hunts and “extream” changes—to describe the various ordered swaps the bells need to make so that they successfully exhaust the circuit and return to the original round, 12345.

The number of the possible orders is given by the factorial, a calculation now expressed in mathematical notation by an exclamation mark. For five bells, the possible number of orders is 5!, or (5 × 4 × 3 × 2 × 1) = 120. The factorial increases at a dramatic rate, and really merits its hysterical punctuation when it reaches 12!, or the number of orders possible on twelve bells: 479,001,600. The size of the numbers generated by the factorial fascinated early writers on change ringing. Richard Duckworth, author of Tintinnalogia (1668), the first book on the practice, wrote about how quickly it had developed, given that “within these Fifty or Sixty years last past, Changes were not known, or thought possible to be Rang.” Now though, he continues, “neither Forty-eight, nor a Hundred, nor Seven-hundred and twenty, nor any Number can confine us; for we can Ring Changes, Ad infinitum.”^[4] Duckworth, whose book explained the structure of change ringing and gave newly devised methods for it, was amazed at how numbers could be made to spread and increase in such a way—but also at how change ringing could corral and comprehend such numerical copiousness.

The Cornish merchant and traveler Peter Mundy, writing in his diary in the middle of the century, also wondered at what he called “the strange operation & mistery of numbers” in the system of change ringing, in which so few units could, when permutated, “amount unto such a prodigious summ.”^[5] Mundy’s journal, which he called, surely knowingly, the “Itinerarium Mundi,” is principally taken up with tales and (endearingly bad) drawings of his travels in Europe, central and east Asia, and India. He wrote about the customs, buildings, and the natural environment he traveled through, including women’s fashions in Bremen, the Indian practice of suttee, and the gruesome punishments given to prisoners in Turkey, Danzig, and Sumatra; he was in Agra when the Taj Mahal was under construction; and he might very well have contributed to the extinction of the dodo, by eating one.

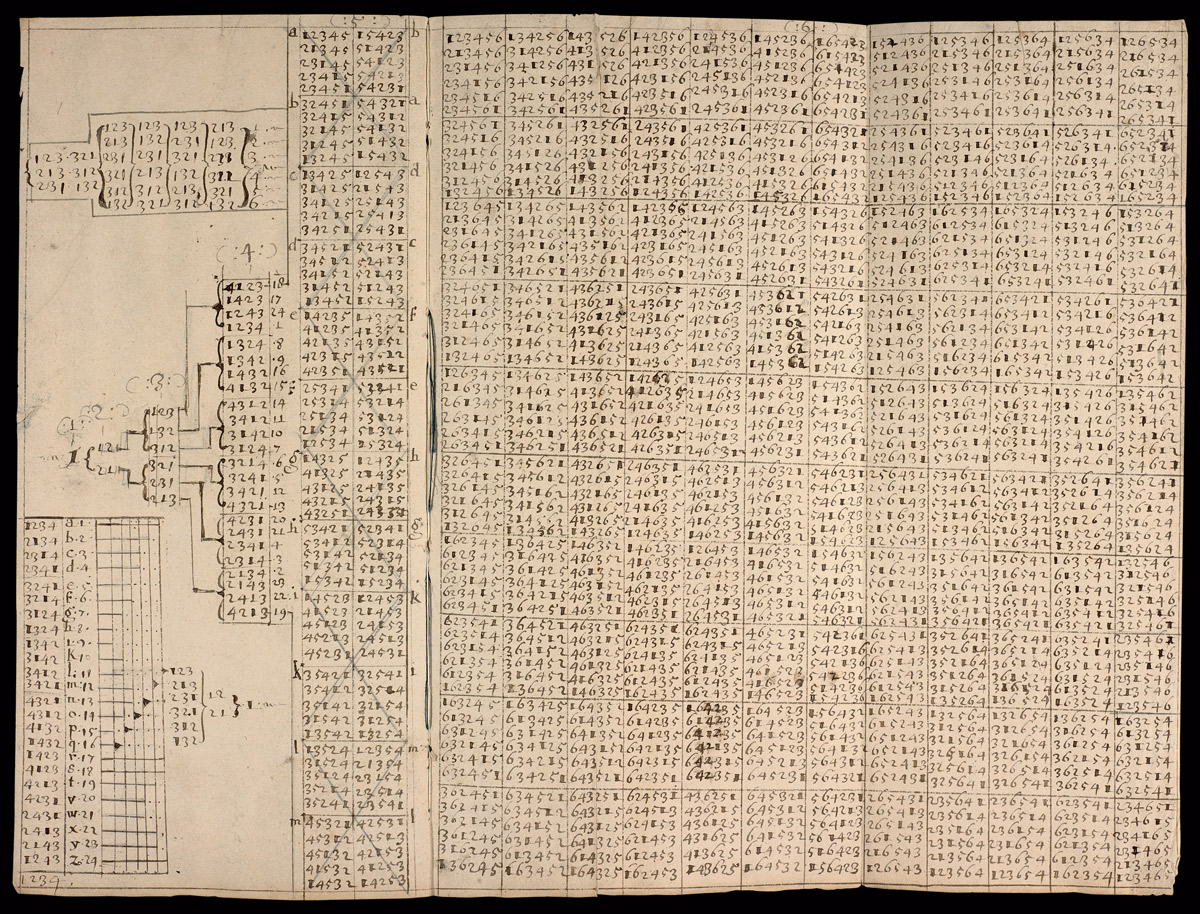
But upon his return to England after several decades, during a visit to London in 1654, Mundy was taken with the new and curious practice of change ringing and wrote about that, too. “The sweet Ringing of our tuneable bells, especially in changes, which [in] my opinion deserves notice,” he writes, “first, for the Art therein to bee observed, (2) their melody, and (3) the singularity of it. Not the like, nor nothing Near, to bee heard in the whole World beeside.”^[6] For Mundy, the “singularity” of the practice came mainly from the numerical profusion that its system produced. On a fold-out piece of paper double the size of his already-large journal, he wrote out all changes possible on three, four and five bells, up to the 720 changes on six bells: the numbers 1–6 written out, painstakingly and compulsively, over and over again until all their permutations had been exhausted.

Change ringing was based on the factorial—an exhilarating, if blunt, form of multiplication—so it did have a basis in mathematical calculation. Indeed, the operations of change ringing permutations can be explained by the branch of algebra known as group theory, which was only codified in the nineteenth century. But for all those copious rows of digits that Mundy wrote out, the numbers of change-ringing notation don’t really need to be numbers. They don’t combine in any arithmetical way and are only used because each digit is unique, but equal; 5 is worth no more and no less than 1. These are numbers that might just as well be shapes, or fruit, or—perhaps—letters. The form of change ringing is, after all, a series of anagrams; the obsessive permutation of the row can be explained by George Puttenham’s description of an anagram, in his influential poetry manual The Arte of English Poesie (1589), that is “to breed one word out of another not altering any letter nor the number of them, but onely transposing of the same.”^[7]

Peter Mundy capitalized on the breeding, generative power of the anagram and turned to letters and to language in order to comprehend the massive numbers immanent in change ringing’s permutations. Mundy seems to have realized that not only are there twenty-four ways of permutating the order of four bells (that is, 4! = 4 × 3 × 2 × 1 = 24) but also that there are, potentially, 24! ways of ordering these twenty-four rows—24! ways of permutating the permutations, if you like. If the factorial of numbers up to six could fill that huge page with his handwritten digits, how much paper might 24! take up? Mundy saw an immediate connection between these unwieldy numbers and the letters of the alphabet. The early modern English alphabet didn’t distinguish between i and j, or u and v, so it had twenty-four letters: a perfect fit. He wrote out all twenty-four permutations possible on four bells, and assigned each permutation (1234, for example, or 2134) to a different letter.

Mundy imagined these permutations of twenty-four letters printed in a series of books. He postulated that each book would contain five hundred leaves, so a thousand pages, and each page would contain two columns with twenty-four changes in each. He estimated the dimensions of each book and then calculated the “superficies” (surface area) of the earth. When laid down, the books would cover, he concluded, an area 754 times greater than that of the surface of the “earth and seas”:

the said number of books wold not be conteyned in 754 such worlds as these if they were laid one by one, butt if so bee they were to be heaped all uppon one world close packed then would the heap bee 188 ½ foote round about or 754000 bookes one uppon another over the whole world imagine’d to be dry land.^[8]

In Mundy’s calculations, the books, covering “754 such worlds,” would smother land and sea with pages and pages of meaningless words. In fact, Mundy’s calculations were off: he made a mistake in his multiplication of 23!, so his final total is wrong, but the application of his numbers is more important than their accuracy. He framed his calculation in terms of a vastness he could begin to comprehend: the book in which these notes are written also contain his diary entries written while he himself was covering the surface of the globe.

In the prefatory essay to his Campanalogia (1677), the second book to be published on change ringing and which, like Tintinnalogia, functioned as a manual for the practice, Fabian Stedman also imagines the permutated bells as letters and thinks about the possibilities they might suggest for language. “If we consider the multitude of different words, wherewith we express ourselves in Speech,” he writes, “it may be thought almost impossible that such numbers should arise out of twenty four Letters; yet this Art of variation will produce much more incredible effects.”^[9] Change ringing opened up Stedman’s imagination to the “art of Variation” generated by the operation of the factorial.

Like Mundy, Stedman displays wonder at the vast numbers produced by the combination of things into larger and more varied objects, but his experiment, though similar, was even more complicated than his predecessor’s. Stedman also uses twenty-four bells as a starting-point to extrapolate into language and uses not just permutation but combination, too: the order of the letters are still permutated, but they do not all have to be present every time. Whereas Mundy imagined the resulting numbers (or words) printed out, Stedman wonders how long it would take people to say each word out loud, guessing that

the infinite numbers of them [words] would not permit a Million of men to effect it in some thousand of years: it would be evident, that there is no word or syllable in any language or speech in the world, which can be exprest with the character of our Alphabet, but might be found literatim and entire therein, and more by many thousands of Millions than can be pronounced, or that ever were made use of in any language.^[10]

Stedman’s is a truly optimistic linguistic experiment. He claims (albeit Eurocentrically) that his combinations include every word and syllable “in any language or speech in the world”—and he has also managed to catch words that are not words, or not yet words: currently meaningless collections of letters that represent not gibberish, but potential. In Stedman’s calculations, bells speak: not the signaling language of their old uses, but a language of permutated units with dizzyingly vast numbers of possibilities.

Both Stedman and Mundy start with the very real ringing of very loud bells, but each quickly accelerates into the imaginary and unringable, performing a drastic abstraction of the bells and their sounds. The number of bells that Stedman and Mundy discuss is unrealistic: because of the mechanisms needed to hang bells for change ringing, there would never be twenty-four bells in a church tower (even today the maximum is almost always twelve, and eight was the most that one was likely to find in the seventeenth century). And although the goal of change ringing’s system was to exhaust all possible changes, the physical constraints and demands on the ringers were such that it was not really possible to ring the complete circuit on any more than seven bells—the possible combinations presented by eight bells (40,320 changes) would, using Stedman’s estimate that ringers could ring 2.4 bells per second, have taken over thirty-seven hours to fully work through.

But the excessive notation imagined by these theorists of change ringing was never really part of the practice. While change ringers must understand the shape of the particular method they are ringing, they do not follow written notation for each and every change. Nor do they memorize the individual changes. Rather, the practice relies on the ringers internalizing the patterns of the method, perhaps by looking at notation that shorthands the whole method, showing only the key moments at which the permutations change course in order to exhaust all the possible orders. Ringers know principally by doing: they anticipate when two bells will have to swap places in the following round, and they feel their way as a group through the ringing of all the orders of the rows. Change ringing’s linguistic potential may have been exploited by Stedman and Mundy, but in the bell-tower it is a sweaty, communal, and profoundly corporeal activity.

Ringers have to work as one body: all performing the same action, like rowers in a boat, but—unlike rowers—not at the same time, with each ringer ringing their individual bell as a part of the whole. That enthusiastic glass-headed ringing that Paul Hentzner described became by 1700 a highly regulated, communal recreation—and as the complexities of change ringing increased, with more bells being rung, and greater sophistication in the patterning of the different methods, the practice became more widespread as well. During the Restoration, and to the end of the seventeenth century, many new societies sprung up to practice and to further change ringing. Some, like the London Western Green Caps, drew their members from apprentices and other young workingmen; others, such as the Esquire Youths, had members of the nobility and gentry among their number, as the society’s name suggests. Bell-ringing was no longer something done by just the loutish lower class: its intriguing system had made it popular as an organized leisure activity among all sorts of people. The strict rules of change ringing’s system were reflected in the written orders to which ringing societies demanded their members agree: the Esquire Youths, for instance, had to swear to rules in two categories: “order in our Arte and exercise or manner in our behaviour & deportm[en]t.”^[11]

Rules governed the permutations, and they governed behavior; change ringing was an activity bound by orders of all kinds. What happened, amid all these rules, to the sound the bells actually made? The urge for order certainly didn’t preclude musicality: Mundy, after all, praised the “melody” of change ringing, as well as its “Art.” It was important that the bells be in tune with one another; ringers must “understand the Tuning of Bells,” cautioned Richard Duckworth, “for what is a Musitian, unless he can Tune his Instrument, although he plays never so well?”^[12] Nevertheless, musical considerations do not seem to have been very important in the drive for permutational exhaustion. The music historian Charles Burney wrote about change ringing in 1789, and particularly about the description of the practice he found in Tintinnalogia. While Burney praised the way in which Duckworth’s book gave “every possible change in the arrangement of Diatonic sounds, from 2 to 12” and “the wonderful variety which the changes in bells afford to melody,” it was precisely the profusion of such changes—the thoroughness of its anagrams, which Stedman and Mundy found so seductive—that rendered the practice ultimately unmusical. “It must not,” Burney continues, “be imagined that all the changes … would be equally agreeable, or even practicable. … [I]t is extraordinary, that melody has not been consulted in the choice of changes: there seems a mechanical order and succession in them all, without the least idea of selecting such as are most melodious and agreeable.”^[13] For Burney, change ringing fails as music because there is no selection, no “choice of changes”: but it was precisely that all changes are present and neatly worked through that was, for Stedman and Mundy, the beauty of the system.

To perceive and understand the totalizing system of this “mechanical order and succession,” listeners of change ringing have to make their ears work hard. The antiquarian Thomas Hearne, Assistant Keeper of the Bodleian Library in the early eighteenth century, was an expert on the practice; he wrote in his diary about how he would walk around Oxford, listening to the ringers ring. His understanding of change ringing was so precise that he could hear when the ringers made a mistake—that is, when they got the pattern wrong and rung a row out of sequence, or repeated a row that they had already rung. This required an intense kind of listening: on one occasion he admitted, “I do not know that I ever gave greater attention to anything in my life.”^[14] This is mathematical listening: a sort of deep aural counting in which those rows of numbers are somehow heard, and understood.

This consideration that change ringing demands of its listeners suggests it to be an ancestor of a much later kind of music: the twelve-tone compositions of Arnold Schoenberg, Anton Webern, and later adherents of serialism. Twelve-tone music is, like change ringing, all anagram: all twelve notes of the chromatic scale must be played, and the order in which they are played must be varied every time according to strict rules. Theodor Adorno, a fan of Schoenberg’s twelve-tone music, wrote that “all that can actually be heard [in these compositions] is that the constraint of the system prevails.”^[15] There is no evidence that Schoenberg or Adorno knew about change ringing, but Adorno’s remark, which seems to answer both Burney and Hearne, might well be applied to its tumbling sound.

Although few people listened as carefully as did Thomas Hearne, the sheer quantity, and the resulting length, of change ringing’s profuse permutations began to gather crowds. In the eighteenth century, large audiences would stand and listen to bell-ringers’ feats of endurance as they competed—for four hours, or more—for trophies of gloves or shirts, or just for the glory. The term peal began to apply to a set of at least 5,040 changes; plaques were put up in ringing chambers to commemorate feats of ringing that achieved these giant numbers. And the profile of the practice shifted: over the course of the eighteenth century, ringing became, once more, largely the preserve of men from the “lower orders,” with far fewer members of the gentry taking part.

Addison’s dumbbell, competitive ringing, Burney’s contempt: by the middle of the eighteenth century, change ringing had changed. The kind of exhaustion that bells offered was not just abstract and permutational but also physical and, though still immensely popular, change ringing was no longer the intellectual curiosity it had been to Mundy and Stedman. The authors of a successor to Stedman’s book, published in 1702, confided that “it will be very improper, and not in the least to our purpose to stuff this Treatise full of unnecessary and useless Examples of the Variation of Numbers.”^[16] But it was just that kind of example that had caught the interest of the early writers on change ringing, and their enthusiasm for the conquering of numbers had in turn driven the development of the practice. Change ringing emptied church bells of their individual sonic characteristics and read them as numbers or as words; it abstracted them so far from the aural and material that they could be transformed into silenced pieces of exercise equipment for Mr. Spectator to huff away with in the quiet and solitude of his London rooms.

See press about “Campanologomania” on Arts & Letters Daily.